New Extended Thin Sheet Approximation for  Geodynamic Applications - II. 2D examples

             Paper  aims to demonstrate the potential of the ETSA by applying it to 2D examples. The system of equations governing the ETSA is reduced to 2D and tested on problems involving one and two layer systems of Newtonean viscous materials. The application of ETSA in each case includes (1) setting boundary conditions, (2) completion of equations by evaluation of coefficients, (3) comparison of equations with governing equations of existing thin sheet approximations, and (4) linear analyses of small perturbations and determination of their dominant wavelengths.
            A set of computer codes for linear analyses of both ETSA and exact equations is developed to investigate the accuracy, analytical behaviour and robustness of ETSA.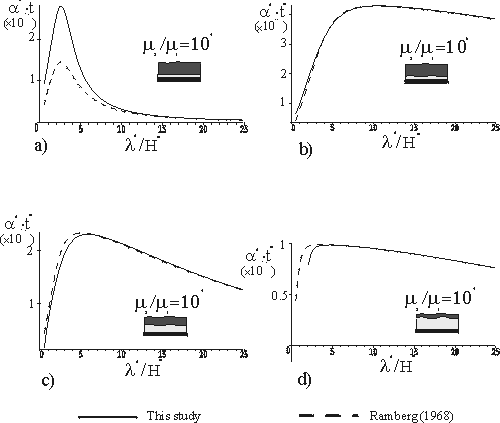
            Linear analyses compare well with exact analytical solutions over a wide range of wavelengths for modelling isostatic adjustment, Rayleigh-Taylor instabilities (results are shown on Fig.: for different viscosity profiles and  thicknesses of layers) and the development of instabilities due to lateral compression and extension. The accuracy of the results provided by ETSA is surprisingly high. Accurate instability spectra defined by linear analyses are obtained by our long-wavelength-approximation, even at short wavelengths.
            It is shown that most previous approaches can be derived by simplification of an extended system under specified boundary conditions. Analyses of instabilities shows that the ETSA can be applied to geodynamic problems that were not tractable to previous thin sheet approximations. Only buckling could previously be handled by the TP approach. The development of other mechanical instabilities was not possible with previous thin sheet models, even where the competence contrast was high and the dominant wavelengths were much longer than the thickness of the thin sheet.