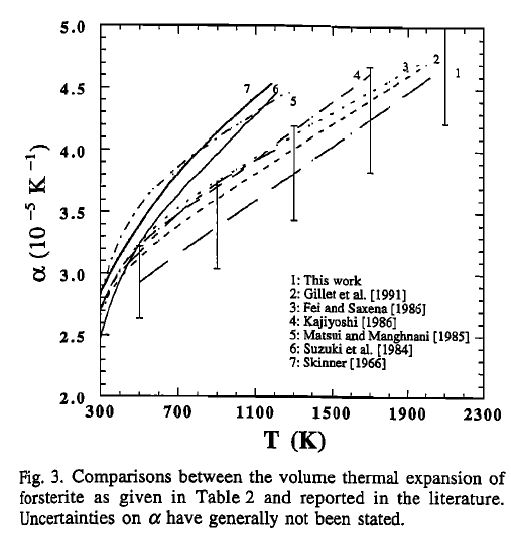
Comments concerning value of the thermal expansivity of the mantle based on measurements of the coefficient of thermal expansion of forsterite and other mantle minerals (October 2010)

Chris Beaumont

Conclusion….probable best choice for an average value is α = 3.5 10 ^-5 K ^-1
2013-01-29:
On reviewing these notes, I think we should use 3.7 x 10 ^-5 for all mantle ....lithospheric and sublithospheric. Later, we can make alpha increase linearly with temperature. Because values of 4.0 can probably be justified at high temperatures

GEOPHYSICAL RESEARCH LETTERS VO L.2 3,NO.1 0, P AGES 1143-1146 ,1996

Thermal expansion of forsterite up to the melting point

M.A. Bouhifd, D. Andfault, G .Fiquet and P .Richelt

Abstract. As determined from powderX -ray diffraction

experiments with synchrotron radiation, the thermal

expansion coefficient of forsterite increases smoothly from

2.8 to 4 .5 K ^-1 from 400K to 2160K . No anomalous increases

of the cell parameters are observed near the melting point. The

consistency between the observed and calculated value of the

initial slope of the melting curve of forsterite suggest that

defects do not make a large contribution to thermal expansion

near the melting point. Along with previous results, the new

data confirm the influence of anharmonicity on the high temperature

heat capacity of forsterite and indicate that both

the Gruneisen parameter and αK_T (α = thermal expansion

coefficient, K_T = bulk modulus ) have nearly constant values at

high temperatures.

--------------------------------------------------------------------------------------------------------------------

Earth and Planetary Science Letters 233 (2005) 337– 349

Thermal structure of oceanic and continental lithosphere

Dan McKenzie , James Jackson, Keith Priestley

Institute of Theoretical Geophysics, Bullard Labs, Madingley Road, Cambridge CB3 0EZ, U.K.

Received 3 September 2004; received in revised form 1 February 2005; accepted 10 February 2005

Available online 8 April 2005

Editor: V. Courtillot

Abstract

Recent studies of the focal depths of earthquakes in old continental lithosphere have shown that they are almost entirely confined to the crust. Except where recent subduction of oceanic lithosphere is likely to have occurred, no earthquakes with a magnitude of N5.5 have yet been located beneath the Moho. In contrast, in oceanic lithosphere earthquakes commonly occur within the mantle. The principal control on whether or not deformation occurs by brittle failure has long been believed to be temperature. We re-examine the thermal models of both oceans and shields. Taking account of the temperature dependence of the thermal conductivity lowers the temperature within the oceanic lithosphere. Except beneath the outer rises of trenches, where the strain rates are large, intraplate oceanic earthquakes are confined to regions cooler than 600 8C. In continental regions most earthquakes occur in the mobile belts that surround Archaean cratons, where the crust is as thick as 50–60 km. Recent studies, of the Canadian Shield in particular, have shown that radiogenic heating is not as concentrated at shallow depths as was previously believed. Taking account of both these effects and the temperature dependence of the thermal conductivity increases the Moho temperatures, which can exceed 600degC, and produces geotherms that agree well with pressure and temperature estimates from nodule suites from kimberlites. Therefore the mechanical behaviour of oceanic and continental upper mantle appears to depend on temperature alone, and there is as yet no convincing evidence for any compositional effects.

Quote from McKenzie et al

Bouhifd et al. [25] (material above) made careful

measurements of a(T) from 599 to 2100 K and found

an increase of almost a factor of two. They show that

their results agree with those of several other groups

Bouhifd et al. parameterise their observations using

α (T) = 1/ρ x dρ/dT = α₀ + α₁ T

α₀ = 2.832 x10^-5

α₁ = 3.79 x 10^-8

Integration gives

ρ(T) = ρ₀ exp(- [α₀ (T-T₀) + α₁(T² – T₀²)/2])

where T₀ =273 K, and ρ₀ =3330 k/m³

Bouhifd et al., 1996

----------------------------------------------------------------------------------

Earth and Planetary Science Letters 257 (2007) 343–349

Effective thermal expansivity of Maxwellian oceanic lithosphere

Jun Korenaga ⁎

Department of Geology and Geophysics, Yale University, P.O. Box 208109, New Haven, CT 06520-8109, USA

Received 25 July 2006; received in revised form 23 February 2007; accepted 4 March 2007

Editor: R.D. van der Hilst

Available online 12 March 2007

Abstract

The thermal expansivity of oceanic lithosphere is a key mineral physics parameter that controls the rate of seafloor subsidence. Because of strongly temperature-dependent mantle rheology, effective expansivity for lithosphere as a whole could be substantially lower than indicated by mineral physics data. Viscoelastic modeling indicates that this reduction in expansivity could be as high as ∼15–30% for a plausible range of mantle viscosity. Though brittle relaxation by thermal cracking is likely to alleviate this reduction, tension cracking results in fractured lithosphere with finite crack porosity, thereby prohibiting the recovery of fullyequilibrated density. Even with complete brittle relaxation, effective thermal expansivity could still be lower by up to ∼20%.

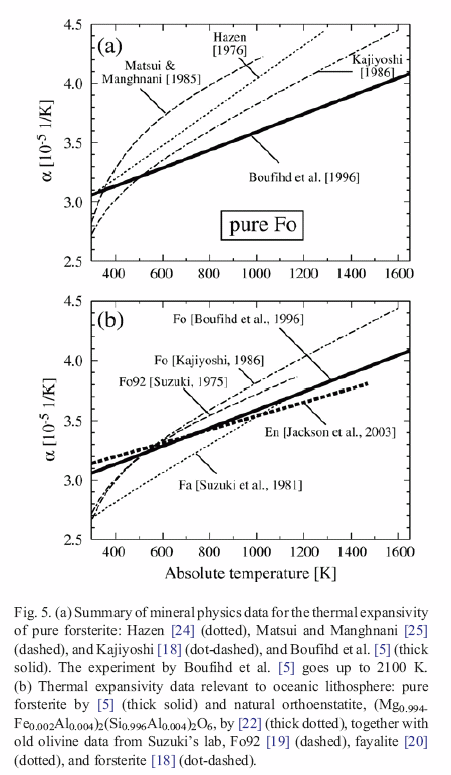
Quote from Korenga…..

Traditionally, the experimental

determination of thermal expansivity tends to

suffer from relatively large uncertainty (partly due to

limited temperature range investigated), and this

viscoelastic effect might be viewed as yet another

source of uncertainty. However, more reliable data have

been emerging in the last decade, and at least for pure

forsterite, its thermal expansivity appears to be firmly

established [5] (Fig. 5a); α is ∼3.1×10−5 K−1 at room

temperature and ∼4.0×10−5 K−1 at 1300 °C. Of

course, the mantle is not made of pure forsterite, and

thermal expansivity for realistic mantle composition is

still somewhat uncertain because we do not have all of

necessary (high-quality) expansivity data. For oceanic

lithosphere, the following two issues are important: (1)

the effect of fayalite component for the expansivity of

olivine, and (2) the thermal expansivity of orthopyroxene.

The expansivity data of fayalite comparable to [5]

does not exist at the moment, but by comparing old data

for pure forsterite [18], Fo92 [19], and pure fayalite [20],

all from the same laboratory (Fig. 5b), it may be

reasonable to expect that the thermal expansivity of

mantle olivine (Fo90–92 for oceanic lithosphere) would

be different from that of pure forsterite by no more than

a few percent. As clinopyroxene is preferentially

consumed by melting, the second most abundant

mineral in oceanic lithosphere is orthopyroxene [21];

olivine and orthopyroxene together constitute about

90% of depleted lithosphere. Just like the case for

olivine, the expansivity data for orthopyroxene have

been controversial, but the most recent experiment [22]

indicates that olivine and orthopyroxene may actually

share a similar thermal expansivity (Fig. 5b). As far as

mineral physics is concerned, therefore, the thermal

expansivity of oceanic lithosphere is now reasonably

well understood and could be approximated as that of

pure forsterite.

The effect of viscous relaxation suggested by this

study is thus not trivial, and more importantly, it systematically

lowers the apparent thermal expansivity. It is

interesting to note that α of 3×10−5 K−1 has long been

a standard value for the upper mantle in geophysical

studies [17], even though in the past three decades

mineral physics data for forsterite have persistently

indicated this value is only appropriate near the room

temperature condition. The long-standing preference to

3×10−5 K−1 may stem from the analysis of seafloor

subsidence. Though their estimates are subject to large

uncertainty, Parsons and Sclater [7] and Stein and Stein

[8] both show that this value is nonetheless the best-fit

value given the observed seafloor subsidence. Incomplete

thermal contraction due to temperature-dependent

viscosity may explain this low expansivity.

To sum, the effective thermal expansivity of oceanic

lithosphere is a function of mantle rheology. Because the

effect of viscoelastic response is shown to be nearly

time-independent, it can be concisely represented by a

single time-averaged reduction factor α¯eff /α. A bound

for possible brittle relaxation can also be calculated by

assuming the density of infilling material. The simplicity

of this result should allow us to easily simulate

effective expansivity in the calculation of heat conduction

and corresponding subsidence. It will be shown

elsewhere [23] that the concept of effective thermal

expansivity is essential to understand the origin of depth

anomalies at old ocean floor

----------------------------------------------------------------------------------------------------------------

Physics of the Earth and Planetary Interiors 174 (2009) 86–92

Thermal expansion of forsterite at high pressures determined by in

situ X-ray diffraction: The adiabatic geotherm in the upper mantle

Tomoo Katsura a,∗, Anton Shatskiya, M.A. Geeth M. Manthilakea, Shuangmeng Zhaia,

Hiroshi Fukui a,b, Daisuke Yamazakia, Takuya Matsuzakia, Akira Yonedaa, Eiji Itoa,

Akira Kuwatac, Akihiro Uedac, Akefumi Nozawad, Ken-ichi Funakoshid

Volumes of forsteritewere measured by in situ X-ray diffraction at simultaneously high pressures and high temperatures up to 14 GPa and 1900K in a Kawai-type multi-anvil apparatus. By fitting the present data to the Mie-Grüneisen-Debye thermal equation of state with the results of the previous reliable studies, we obtained _0 = 1.31(1) and q = 3.2(1). The logarithmic volume dependence of the thermal expansion coefficientwas found to be ıT = 8.4(2). The average temperature derivative of the isothermal bulk modulus in the investigated pressure–temperature rangeswas found to be (∂KT/∂T)P =−0.028(1) GPa/K. The isobaric heat capacity and thermal pressure at high pressure and high temperature were estimated using the present volume data. The adiabatic geotherm at the top and base of the uppermost mantle are 0.60(2) and 0.34(2) K/km. The temperature estimated from the 410-km discontinuity depth and the olivine-wadsleyite transition pressure suggests a mantle potential temperature of 1520±40 K.

3.4. Thermal expansion coefficient at high pressures

Values of the thermal expansion coefficient, ˛, can be obtained

by comparing the Mie-Grüneisen-Debye EoS (Eq. (3)–(6)) with the

Birch-Murnaghan EoS (Eq. (2)) for varying temperature. Values of

˛ at 0, 5, 10, and 15 GPa are plotted against temperature in Fig. 6.

At ambient pressure, ˛ greatly increases with temperature at temperatures

higher than 1000 K. At high pressures, ˛ almost linearly

increases with increasing temperature at high temperatures. The

anharmonic effects are significantly suppressed even by small pressures.

Fosterite…Katsura 2009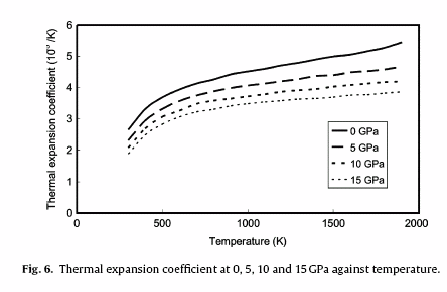

Note this takes into account the pressure effect on the thermal expansivity. It reduces the high temperature increase somewhat

My preliminary conclusion is that α (mantle)……probably both lithosphere and sublithospheric mantle to depth of 440Km is > 3.1 10^-5 (It certainly is not 2 10^-5).

We have 2 options…1) Introduce a temperature dependent α

2) Use an average value representative of the range overwhich mantle

temperatures range in the models. Such a value would be approximately 3.5 10^-5

We need to do more work on this to see if crustal values of thermal expansivity require a temperature dependent formulation.

I believe all of this is important in general…we need to refine parameter values.

It is most important for models with lateral gravitational flow of mantle…e.g. cratonic mantle because density variations caused by thermal expansion are traded off in a sensitive way against chemical depletion.